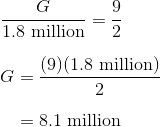This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 6 a shot before reading this!

6.18: State Populations, Pt. 2

Let’s use G to denote Georgia’s population, and W to denote that of West Virginia. In those terms, the problem supplies us with two pieces of information:

Plugging our value for W into the equation gives us

Because 8.0 < 8.1 < 9.9, Georgia belongs to population category E — which, somewhat confusingly, corresponds to answer choice (D).

Math Review Reference

For more on this topic, see the following sections of the GRE Math Review:

• 1.6: Ratio (p. 9)
• 4.1: Graphical Methods for Describing Data (pp. 62-64)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 6 a shot before reading this!

6.17: State Populations, Pt. 1

Turn the page, and we’re back to the chart block. This set of problems is a little more straightforward than those in Section 5, mainly because there’s only one data graphic to deal with. For this problem, a simple and effective approach would be to add up the “Number of States” column for each of the first five categories:

There is, however, an alternate route that some students find faster and simpler. The chart tells us (or, depending on where you’re from, reminds us) that there were 50 states in the USA during the year in question. So instead of counting up, we can start with 50 and subtract the three categories excluded by the problem:

Either way, we arrive at 43 states, which corresponds to answer choice (B).

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 4.1: Graphical Methods for Describing Data (pp. 62-64)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 6 a shot before reading this!

6.16: A Slight Miscalculation

To solve this problem, we need to understand what effect Chris’s mistake had on the result of his calculation. 2,073, the factor that Chris entered into his calculator, is 1,000 times the intended factor of 2.073. So the product will be 1,000 times too large. Consequently, the correct answers will be operations that make the product smaller.

Of the four answer choices, only (A) (“multiply by 0.001”) and (D) (“divide by 1,000”) meet this criterion: either operation would cancel out the erroneous factor of 1,000. Answers (B) and (D) actually make the product even larger, amplifying the original error.

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 1.5: Real Numbers (pp. 7-8)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 6 a shot before reading this!

6.15: Counting Integers

This problem is an illustration of the multiplication principle (also known as the rule of product), which states:

If you have two independent choices to make, one with x different possibilities and another with y different possibilities, then there are xy possible pairs to choose from.

If you have 5 choices of entrée and 3 choices of dessert on a dinner menu, then you have 15 possible choices for your meal. Similarly, if you choose one of 7 ice cream flavors and one of 4 toppings, then you have 28 possible arrangements to choose from. (For some mysterious yet delightful reason, all of the classic examples of this principle involve food.)

In this problem, we apply the multiplication principle by

• figuring out how many possibilities there are for the tens digit (x)
• figuring out how many possibilities there are for the units digit (y)
• multiplying together x and y to get the overall number of possibilities

The tens digit, we’re told, must be greater than 6. Given that restriction, there are 3 possibilities:

{7, 8, 9}

The units digit has to be less than 4, which leaves us with 4 possibilities (don’t forget zero!):

{0, 1, 2, 3}

3 possibilities for the tens digit and 4 for the units digit makes

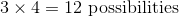
which corresponds to answer (D). Specifically, the possible integers are

{70, 71, 72, 73, 80, 81, 82, 83, 90, 91, 92, 93}

but you clearly don’t need to list the possibilities to get the problem right. In fact, other problems of this type may make it impractical to enumerate the possibilities directly, which is why (apart from saving time) the multiplication principle is so important. (Consider what this problem would be like if it asked about four-digit integers: listing out all of the valid numbers would be extremely unwieldy and time-consuming, but the multiplication principle would still work just fine.)

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 4.3: Counting Methods (p. 75)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 6 a shot before reading this!

6.14: Area of a Triangle

This is a little more abstract than the usual GRE triangle problems (e.g., 5.24), but it isn’t any more complicated. All we need to do is recall the triangle area formula:

and replace b with 2h:

Then, we simplify (by combining like terms) to get:

which corresponds to answer choice (C).

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 3.3: Triangles (pp. 47-49)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 6 a shot before reading this!

6.13: Property Tax

This is another percent-difference problem, similar to 5.19.  Last time, just to refresh your memory, we described the situation in terms of percent change, even though it was actually two different types of taxes we were comparing. Here’s the formula we used.

We can take a similar approach here. Because we’re asked to find our answer in terms of Patricia’s property tax, her tax will be the base, or denominator, in our calculations. The “change” will be the difference between Patricia’s and Steve’s respective property taxes. We’ll call Patricia’s tax P and Steve’s tax S.

But before we can plug those figures into the formula, we need to figure out the value of P. We know S and we know the difference between the two, so we can use those two values together to solve for P:

Now we just plug in P as our denominator, and the difference P – S (which we already know is $140) as our numerator:

Steve’s property tax is about 6.7 percent less than Patricia’s, so the answer is (A).

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 1.7: Percent (pp. 9-12)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 6 a shot before reading this!

6.12: The Midpoint Formula

The GRE Math Review doesn’t actually discuss the midpoint formula, but it’s a fundamental coordinate-geometry fact and ought to be committed to memory. You may not need it to get a problem right on the GRE, but knowing it will certainly save you some time if you encounter a question like 6.12.

Here’s the standard form of the formula:

And here’s a prose translation:

To find the midpoint M of two points on an xy-plane, we take the averages, respectively, of the x– and y– coordinates. The x-coordinate of M is the average of the x-coordinates; the y-coordinate of M is the average of the y-coordinates. 

To find the midpoint of R and S, let R be point 1 and S be point 2. Then:

which corresponds to answer choice (C).

Note that this is yet another coordinate-geometry problem we solved without diagramming. I probably seem like I’m harping on this point, but I’ve had more than one student approach this problem by methodically sketching out a pair of xy-axes, then locating R and S on the coordinate plane. This kind of detail is fine if you’re submitting homework for a persnickety TA, but it’s counterproductive on the GRE, where time is of the essence.

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 2.8: Coordinate Geometry (pp. 30-32)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 6 a shot before reading this!

6.11: Convention Goers

The theme of this problem is “percentages of percentages.” We could use a table as in problems 5.9 and 5.21, but that’s overkill here, because the problem is so direct. If 32% of the convention goers came from Asia, and 45% of that subgroup are women, then women from Asia constitute

of the overall group of convention-goers. That’s all there is to it, but don’t worry: we’ll get more practice with percentages in problem 6.13.

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 1.7: Percent (pp. 9-12)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 6 a shot before reading this!

6.10: Dueling Copiers

This is a special kind of algebra problem called a work-rate problem. There is a basic procedure for these that we can follow every time. It boils down to two steps:

• Figure out the work rate of each machine (worker, factory, etc.).
• Apply those rates to solve for the target quantity.

In this problem, we have enough information to calculate each machine’s work rate directly:

What we really want to know, however, is the difference in the output of the two machines if each one runs for a specified amount of time:

So we plug in the times (which the problem provides) along with the rates we calculated:

The difference in output is

so the correct answer is (B).

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 2.7: Applications (pp. 25-27, see esp. Example 2.7.4)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 6 a shot before reading this!

6.9: Prime Factors

Another page, another great plug-in opportunity. For any given n, it will only take us a few steps to evaluate Quantities A and B. Moreover, plugging in even one value for n will allow us to clear away two of the four answers. At that point, even if we run out of time and have to guess, we’ve doubled our chance of getting the problem right.

First, just to clear up a possible misconception: different prime factors means no duplicates are allowed. The prime factorization of 60 is

so 60 has 3 different prime factors: 2, 3, and 5.

Let’s try out some different values of n. First, let n = 1, the smallest integer allowed by the problem:

When n = 1, the number of different prime factors of 9n (Qty. A) is equal to the number of different prime factors of 8n (Qty. B). This means we can rule out answers (A) and (B).

For our next plug-in, let’s try n = 2.

This time, Qty. A (number of different prime factors of 9n) is greater than Qty. B (number of different prime factors of 8n). Because the quantities are sometimes equal, and sometimes unequal, we choose answer (D) for “depends on the variable.”

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 1.1: Integers (pp. 1-2)