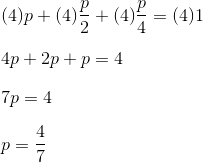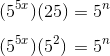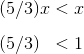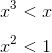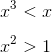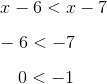This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 5 a shot before reading this!

5.23: A Certain Experiment

Unlike problem 5.9, which was merely “probability-flavored,” this problem actually tests your knowledge of a few basic probability properties. The problem stem gives us two key facts about the outcomes of the experiment:

1. They are collectively exhaustive (at least one of the three must occur).
2. They are mutually exclusive (only one of the three can occur in a given trial).

Because the outcomes are collectively exhaustive, the sum of their probabilities must be at least 1. Because the outcomes are mutually exclusive, the sum of their probabilities can be at most 1. Putting the two together, we arrive at a helpful rule:

If a set of events is both collectively exhaustive and mutually exclusive, the probabilities of the events sum to 1.

In this problem, the rule translates to the following equation:

at which point we’ve reduced a probability problem to an algebra problem. (On the GRE, this is always a step forward.) Now we can solve for p directly:

which corresponds to answer choice (D).

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 4.4: Probability (pp. 78-80)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 5 a shot before reading this!

5.22: Factors and Divisibility

Divisibility, factoring, and primes are big themes in GRE arithmetic, but the problems vary widely in difficulty. Unlike algebra and geometry, there are few telltale signs to let you know when a factoring problem is going to be hard, time-consuming, or both. The underlying math is usually pretty simple, but you may have to try a bunch of different cases before you eliminate all of the incorrect answers.

Here, we can start by finding a number that is “divisible by both 8 and 15.” Rather than looking around for such a number, we can manufacture one by multiplying the two factors together:

(8)(15) = 120

Now, we need to see if 120 is divisible by each of the integers in the answer list. (We can do this by inspection, or we can break out the on-screen calculator.) “Divisible” means that if we divide 120 by that integer, the quotient will also be an integer.

Of our five potential divisors, only 24 (B) survives the divisibility test.

In a way, we’ve lucked out here: the first product we tried (120) was sufficient to eliminate all of the wrong answers. If you found this problem just a little too easy, consider the following slightly harder variant:

x is an integer divisible by both 28 and 6. x must also be divisible by which of the following five integers?

{8, 12, 16, 24, 36}

If you try to approach this problem using the same method as in 5.22, what happens? Why? What makes the factor pair {28, 6} different from the pair {8, 15}, and what must be done differently as a result?

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 1.1: Integers (p. 1)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 5 a shot before reading this!

5.21: Judicial Appointees

This problem has some major similarities to 5.9. Both ask us to reason about a group of people based on a set of overlapping characteristics. Because there are two “dimensions” to this problem (gender and minority status), we can even use the same table setup as in 5.9, where the dimensions were sex and age.

Here’s our initial setup, with the target value represented by bold question marks:

Most of the remaining information is given in terms of percentages or fractions. Just as in 5.9, we need to translate the percentages and fractions into whole numbers, since this is the form our answer will have to take. We begin by considering women and minority groups separately:

• (0.30)(180) = 54 appointees were women
• (0.25)(180) = 45 appointees were from minority groups

Here’s what that looks like in chart form:

The next piece of the puzzle is the overlap between women and minority groups:

• (1/9)(54) = 6 appointees were women from minority groups

Adding that fact to our chart allows us to fill in all of the remaining blanks.

At this point, we can see that 87 of the judicial appointees were neither women nor from minority groups. Therefore, the correct answer is (C).

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 4.3: Counting Methods (pp. 73-75)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 5 a shot before reading this!

5.20: You Need Both to Reach the Arrow

At long last, we come to a problem in which both charts are required. Specifically, we need to reason our way from Germany’s total tax revenue (mentioned in the pie chart) to Germany’s total retail gasoline sales (mentioned in the bar graph). The bridge between the two is Germany’s gas tax revenue, which appears in both charts.

First, let’s figure out the dollar amount of Germany’s gas tax revenue, using the pie chart. We know that the gas tax accounts for 20.4% of the total tax revenue, and we know that the total tax revenue is $170 billion. Combining those two, we get

Because the answer is expressed in billions, there’s no benefit to writing out the $170 billion longhand.

Sanity check: Before we move on, notice that the gas tax revenue has to be less than the total retail gasoline sales. This means that our answer (Germany’s total retail gasoline sales) has to be greater than $34.68 billion. We can rule out (A), (B), and (C) without calculating the total retail gasoline sales directly.

To finish the calculation, we go to the bar chart, where we find that Germany’s gas tax revenue is 70.7% of its total retail gasoline sales. That fact, combined with our previous calculation of the gas tax revenue in dollars, is enough to give us a figure for total sales:

Of the answers provided, the closest approximation to total sales is $50 billion, making (E) the correct answer choice.

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 4.1: Graphical Methods for Describing Data (pp. 62-65)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 5 a shot before reading this!

5.19: Percent Comparisons

This problem is best approached in terms of percent change, using the same principles as we’d employ to figure out a percent discount or markup. Following the examples in the Math Review (pp. 11-12), we can write the basic formula for such problems as

We’re instructed to find the “percent less than” the income tax revenue, so the income tax revenue will be our base (the denominator). The numerator will be the difference between the income tax revenue and the gas tax revenue. Putting that all together, we get:

The gasoline tax revenue is 28.7% less than the income tax revenue. Of the answer choices, the closest approximation to this figure is 30% (answer C).

Math Review Reference

For more on this topic, see the following sections of the GRE Math Review:

• 1.7: Percent (pp. 9-12)
• 4.1: Graphical Methods for Describing Data (pp. 64-65)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 5 a shot before reading this!

5.18: Gas Tax Facts

Here’s another one of those “triple true-false” questions. Just as in a Reading Comprehension passage, we have to step through each answer choice and check to see if it’s supported by the data. Moreover, just like a Reading Comprehension question, the devil is in the details: we need to read carefully, so as not to be taken in by tricky wording.

First, let’s consider answer (A). At a glance it might seem that this statement is supported by the bar chart, but look again: the bar chart lists gas tax revenue “as a percent of total retail gasoline sales,” but the statement concerns France’s gas tax revenue “as a percent of total tax revenue.” We don’t have any information about the distribution of France’s total tax revenue — only Germany’s. Answer (A) is therefore incorrect.

How about answer (B)? Again, this statement refers to figures that aren’t actually presented in the graphs, which don’t mention the actual “price per gallon” in any of the nine countries. It may be tempting to assume that gas will be more expensive in Norway, since gas taxes are higher there than in Spain. But there are all sorts of other factors — transport costs, for example — that might affect the per-gallon price in each of the two countries. Because we can’t say how those other factors will influence prices, we reject answer (B) for insufficient evidence.

At this point, we know answer (C) is correct, because we’ve ruled out (A) and (B), and at least one answer is correct in every multiple-choice GRE question. To see why (C) is right, look at the pie chart. Germany’s gas tax revenue is given as 20.4% of the total, and the tobacco tax revenue is 5.6%. Because 20.4/5.6 is greater than 3, the gas tax revenue must be more than triple the tobacco tax revenue.

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 4.1: Graphical Methods for Describing Data (pp. 62-65)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 5 a shot before reading this!

5.17: Median Gas Tax

This is the first problem in the “chart block,” which is like Reading Comprehension for math. The questions in this subsection tend to involve lots of arithmetic and some basic statistics (esp. mean and median). You may also need to employ some simple word-problem algebra, but the emphasis here is on using the charts to find the data requested, then performing simple calculations on that data.

In most cases, the chart block will include two charts or graphs of different kinds. It could be a bar graph and a pie chart (as it is here), or a table and a scatter plot, or some other combination. In any case, an important preliminary step for each question is to figure out which chart(s) are relevant. Easier problems will use data from only one of the charts on the page, but in harder problems, you’ll need to synthesize or compare data from both.

In 5.17, we have it relatively easy: the problem stem itself instructs us to look at the bar graph. There are an odd number of countries represented, so to find the median, all we need to do is arrange the values in order:

{24.1%, 40.0%, 56.9%, 62.0%, 67.6%, 70.0%, 70.7%, 72.7%, 76.8%]

The median, which corresponds to answer choice (A), is underlined above.

In fact, we don’t even need to write down a separate list, because the graph is drawn to scale. Instead, we can read the graph from left to right, stopping when we reach the fifth of the nine values. (On the GRE, data graphics like the ones here are always drawn to scale, as are coordinate planes and number lines. Other geometric figures, however, are not necessarily drawn to scale — a point we’ll revisit in later questions.)

Math Review Reference

For more on this topic, see the following sections of the GRE Math Review:

• 4.1: Graphical Methods for Describing Data (pp. 61-64)
• 4.2: Numerical Methods for Describing Data (p. 69)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 5 a shot before reading this!

5.16: Powers of Five

When it comes to exponents, the GRE is a little like an old Dos Equis commercial: “I don’t always test exponents, but when I do, I prefer to test multiple properties in the same question.” To get started, we observe that 25 is a power of 5 and can be rewritten as 5²:

From there, we can use the product rule to combine exponents with the same base:

The final step involves a rule known as the property of equality of exponential functions, which is introduced (but not named) on p. 18 of the Math Review:

This is true for any positive base (other than 1) and for any integers a and b. Our base here is 5, and both 5x + 2 and n are integers, so this equation meets all of the criteria for applying the rule:

The answer, therefore, is (B). But take a look at answer (D) for a moment. This is the result you’d get if you accidentally used the power rule (which doesn’t apply to this problem) instead of the product rule. Sneaky, right? “Near-miss” answers like this are actually quite common in GRE algebra problems. It’s important, therefore, to be familiar with all the exponent rules given in the Math Review, and to know when to apply each of them.

Math Review Reference

For more on this topic, see the following sections of the GRE Math Review:

• 1.3: Exponents and Roots (p. 5)
• 2.2: Rules of Exponents (pp. 18-20)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 5 a shot before reading this!

5.15: An Inequality Ready Check

This problem is designed to test your comfort level in manipulating inequalities. When they hand you a problem like this, the testmakers are checking to see if you know one rule in particular:

When you multiply or divide both sides of an inequality by a negative number, the direction of the inequality reverses.

As a little warm-up, consider the inequality

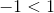
If we multiply both sides by (-1), we have to reverse the sign, or the statement won’t make sense anymore:

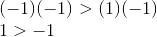
This is important in problem 5.15 because it asks us to consider both positive and negative values of x. We can simplify our task by considering the positive values first, but ultimately we’ll need to deal with the negatives as well. Let’s step through the answer choices one at a time.

Answer Choice A

Let x be any positive number. Then we can divide out the x from both sides as follows:

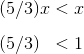
But this is a contradiction, because 5/3 is actually greater than 1. Since assuming that x is positive leads to an incorrect result, we can conclude that inequality (A) has no positive solution. Answer choice (A) is therefore incorrect.

Answer Choice B

Again, we begin by assuming x is positive and dividing out an x to simplify the expression:

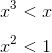
Because x is positive, taking the square root of both sides gives us

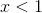
In other words, inequality (B) is true for any positive x less than 1. So answer choice (B) passes our first test. Now what about negative values of x?

Let’s go back to the original inequality, this time assuming that x < 0. Under this new assumption, if we divide out an x from both sides, we have to flip the sign of the inequality.

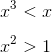
This time around, we don’t even have to take the square root: almost any negative value of x is a solution. (Try x = -2, for example, or x = -10.) Because we can find both positive and negative solutions for the inequality, answer choice (B) is a keeper.

Answer Choice C

That leaves us with one final option to evaluate. This one’s actually a little simpler, because we can subtract out the x rather than dividing or multiplying. When you add or subtract a number from both sides of an inequality, it doesn’t change the sign. Thus, we don’t have to decide in advance whether x is negative or positive.

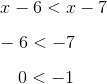
Indeed, it doesn’t end up mattering whether x is negative, positive, or even zero. As shown above, any real value of x leads to a contradiction, meaning that inequality (C) has no real solution at all. We can therefore reject answer (C), leaving (B) as the only correct answer.

A Note on Time Management

As you may have noticed, 5.15 is more time-consuming than most of the other math problems we’ve encountered so far. That’s largely because it requires you to test out a bunch of different cases and see which ones are valid. In other problems, like 5.11 and 5.13, you set up a single equation and solve for a single variable, but in this problem, it’s imperative that you test out each of the answer choices. Even if algebra is your strong suit, “case-by-case” problems like 5.15 may be worth skipping on your first pass through the section. That way, you’ll be sure not to miss any of the faster, easier problems that might appear later on.

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 2.5: Solving Linear Inequalities (pp. 23-24)

This page is part of my unofficial solutions manual to the GRE Paper Practice Book (2e), a free resource available on the ETS website. They publish the questions; I explain the answers. If you haven’t worked through the Practice Book, give Section 5 a shot before reading this!

5.14: An Average Problem

The trick to this problem is recognizing that we can’t solve for x and y separately; there just isn’t enough information. Instead, we have to solve for the sum of x and y and use that to figure out what’s going on in list M.

To find an average, we sum all of the values in a data set, then divide by the number of values in that set:

We can use this formula to find any of these three pieces of information, provided that we have the other two:

• If we know the total and the number of values, we can find the average.
• If we know the average and the total, we can find the number of values.
• If we know the average and the number of values, we can find the total.

That last application is what we need here. For list L, we know the average (10/3) and the number of values (3), so we can solve for the total:

Once we know the total of list L, we can determine the value of x + y:

That, in turn gives us enough information to find the total of list M:

We already know the number of values in M (5), so we can now solve for the average:

In this case, no further simplification was necessary. But remember: on an NE problem, you don’t need to convert your answer to lowest terms unless explicitly instructed to do so. If your answer is equal to the right answer, it is the right answer.

Math Review Reference

For more on this topic, see the following section of the GRE Math Review:

• 4.2: Numerical Methods for Describing Data (pp. 68-69)